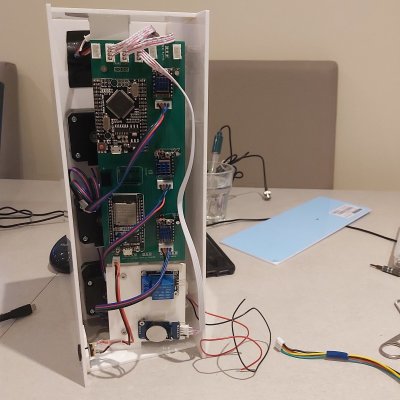
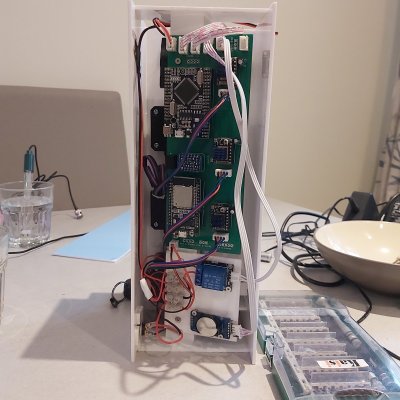
This is a well-tested technology for me now..
Reaction chamber will be 150mm high - this is sufficient to hold 130ml of sample water. The monitor will generally use 100ml sample water. For 100ml sample and alkalinity of 8 DKH the approximate volume of 0.1N hydrochloric acid is 2.9ml. Pumps are able to dose acid with high precision: pumps imperfection will be less than 0.1DKH accuracy.
Main inaccuracy in titration is actually coming from your PH probe precision, not the pumps.

Let me elaborate on the post I replied a few days earlier so as to make it a bit more mathematical for the wider audience:
- the dosing heads I am using utilise 2mm ID / 4mm OD tubing
- such dosing head needs to rotate roughly 5.25 turns to dose 1ml of liquid (how do I know? I ran pump calibration a few days earlier)
- 3ml of 0.1N hydrochloric acid is roughly equivalent to 8 DKH, therefore 0.1DKH is equivalent to 0.0375ml of acid dosed (3.0/[8.0*10]=0.0375)
- Nema 17 motor minimum turn angle (micro-step) is 1.8 degrees (full circle = 360 degrees), therefore it does 360/1.8=200 micro-steps (exactly that number) in a single rotation
- in order to dose the amount of acid equivalent of 0.1 DKH precision, the pump needs to do 0.2 turns (0.0375*5.25 = 0.2) or 40 micro-steps (200*0.2 = 40)
- I can turn the stepper motor by just a single micro-step: this way I would add the amount of hydrochloric acid to achieve 0.003 DKH precision (0.1 DKH / 40)
- in reality you cannot dose liquid below certain amount/precision irrespective of your available technology/equipment (such minimum amount being the volume of a single droplet)
- as an example consider Hanna Alkalinity tester (if you ever used it): you add acid to you 4ml sample volume by adding acid droplet-wise (until the sample changes colour from pink to lavender), a single droplet is probably roughly equivalent to 0.1 DKH and you simply cannot achieve better precision than that however hard you try (unless you manage to use a syringe with thinner needle)
As a summary: the pump dosing precision is 40 times more precise (!!!) than is required to achieve 0.1 DKH precision, but because of "quantization" of dosing restricted by droplet size the real precision is probably around 0.02 DKH accuracy (by my estimates).
Last edited: